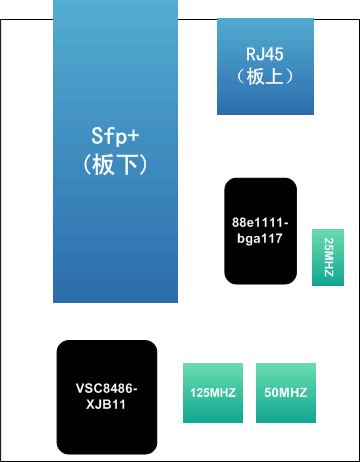
FMC子卡模块学习资料第272篇:1路万兆光纤SFP+和1路千兆网络 FMC子卡模块
本文共 677 字,大约阅读时间需要 2 分钟。
| 1路万兆光纤SFP+和1路千兆网络 FMC子卡模块 | |
|
转载地址:http://iynlz.baihongyu.com/
你可能感兴趣的文章
Mysql学习总结(62)——MySQL连接com.mysql.jdbc.exceptions.jdbc4.CommunicationsException: Communications link问题
查看>>
Mysql学习总结(63)——Mysql数据库架构方案选择与分析
查看>>
Mysql学习总结(64)——Mysql配置文件my.cnf各项参数解读
查看>>
Mysql学习总结(65)——项目实战中常用SQL实践总结
查看>>
Mysql学习总结(66)——设置MYSQL数据库编码为UTF-8
查看>>
Mysql学习总结(67)——MYSQL慢查询日志
查看>>
Mysql学习总结(68)——MYSQL统计每天、每周、每月、每年数据 SQL 总结
查看>>
Mysql学习总结(69)——Mysql EXPLAIN 命令使用总结
查看>>
Mysql学习总结(6)——MySql之ALTER命令用法详细解读
查看>>
Mysql学习总结(70)——MySQL 优化实施方案
查看>>
Mysql学习总结(71)——MySQL 重复记录查询与删除总结
查看>>
Mysql学习总结(71)——数据库介绍(MySQL安装 体系结构、基本管理)再回顾
查看>>
Mysql学习总结(72)——MySQL 开发者开发,设计规范再总结
查看>>
Mysql学习总结(73)——MySQL 查询A表存在B表不存在的数据SQL总结
查看>>
Mysql学习总结(74)——慢SQL!压垮团队的最后一根稻草!
查看>>
Mysql学习总结(75)——并发量大、数据量大的互联网业务数据库设计军规
查看>>
Mysql学习总结(76)——MySQL执行计划(explain)结果含义总结
查看>>
Mysql学习总结(77)——温故Mysql数据库开发核心原则与规范
查看>>
Mysql学习总结(78)——MySQL各版本差异整理
查看>>
Mysql学习总结(79)——MySQL常用函数总结
查看>>